If you ever want to sound foolish during your next television interview on the markets, tell them that the market is approaching a Fibonacci level and will do X when it gets there. It is sort of like doing a DIY network interview and saying the house will have four bedrooms because you are using a 32-ounce hammer.
Both the hammer and Fibonacci are tools used in their respective fields. A hammer is used in carpentry to, among other things, frame a house. A Fibonacci – usually a Fibonacci retracement – is used in investment analysis to frame the market’s recent move.
Obligatory definitions
Here is the section where we have to define what Fibonacci is and we’ll focus on Fibonacci retracements. If you have any interest in who Mr. Fibonacci was, feel free to fire up the Google. It is irrelevant to what we are doing here.
Mr. F’s claim to fame was discovering a mathematical sequence that seems to describe a lot of geometry in the real world from how petals spiral on a flower to how stars spiral in a galaxy. And from that sequence, we get the “golden ratio” or “divine proportion” which somehow describes pleasing and useful relationships in art, music, architecture, and design in general.
The ratio of .618 to 1 comes from a sequence of numbers where any number is the sum of the two that preceded it. Here is it in all its glory:
0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, 610, 987, 1597, 2584, 4181, 6765, 10946, 17711, 28657, 46368, 75025, 121393, 196418, 317811, …
As you can see, the numbers start to get huge. Fortunately, we rarely encounter any above 144.
And even more fortunate, the ratio of any two consecutive numbers settles on .618 fairly quickly. As follows:
0, 1, 0.5, 0.666667, 0.6, 0.625, 0.615385, 0.619048, 0.617647, 0.618182, 0.617978, 0.618056, 0.618026, 0.618037, 0.618033
First, this is the ratio calculated out to the number 610 in the sequence.
Second, and more important, you can see how the ratio oscillates higher and lower as it hones in on the .618 value. Any ratio beyond what is shown above is pretty much just rounding error away from that value.
Voila! the golden ratio!
Fun for math nerds
The ratio has all sorts of neet-o properties. For example, 1 / .618 = 1.618.
And 1 – .618 = .382. Guess what the square root of .382 is? That’s right, .618.
.382 / .618 = .618
You have probably seen these two percentages on the charts and they are roughly equal to the simple percentages traders have used for years, namely 1/3 and 2/3 (or .333 and .667).
Guess what is not a Fibonacci number. 50% or .5. Check out the sequence. It is nowhere to be found. And you cannot concoct it from math-ing 0, 1, and .618, either.
Still, traders do attach meaning to a 50% retracement or pullback and it has wormed its way into analysis using Fibonacci. Don’t fight it, exploit it.
Using this stuff
Now is when we get to dispense with the formalities and use the same experience chart watchers use – fibos. Don’t confuse this with fibbie, which is the FBI. Or fubar, which was what you may be feeling right now trying to digest all this fibo stuff.
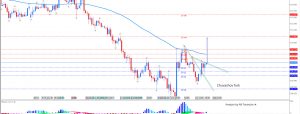
Fire up any charting software package and under the drawing tools section you will see Fibonacci retracements and possibly Fibonacci extensions, time and arcs. They are all just different ways to look at two points on a chart – usually a high and a low – and then draw stuff off of them at specific percentages.
Take a look at this chart of the S&P 500 from 2007 to 2011. Using the basic set of fibo retracements of 38.2%, 50% and 61.8% look how nicely we could project where support and resistance might be.